Determinant of a matrix wikipedia
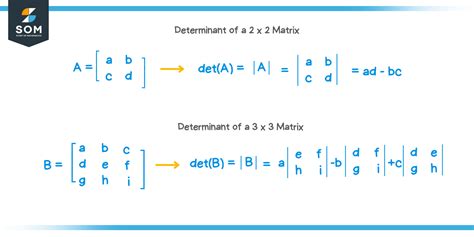
WebOct 11, 2024 · Definition Determinant of Matrix. Let $\mathbf A = \sqbrk a_n$ be a square matrix of order $n$.. That is, let: $\mathbf A = \begin {bmatrix} a_{1 1} & a_{1 2 ... In mathematics, the determinant is a scalar value that is a function of the entries of a square matrix. It characterizes some properties of the matrix and the linear map represented by the matrix. In particular, the determinant is nonzero if and only if the matrix is invertible and the linear map represented by the matrix … See more The determinant of a 2 × 2 matrix $${\displaystyle {\begin{pmatrix}a&b\\c&d\end{pmatrix}}}$$ is denoted either by "det" or by vertical bars around the matrix, and is defined as See more If the matrix entries are real numbers, the matrix A can be used to represent two linear maps: one that maps the standard basis vectors to the rows of A, and one that maps them to the columns of A. In either case, the images of the basis vectors form a See more Eigenvalues and characteristic polynomial The determinant is closely related to two other central concepts in linear algebra, the eigenvalues and the characteristic polynomial of a matrix. Let $${\displaystyle A}$$ be an $${\displaystyle n\times n}$$-matrix with See more Cramer's rule Determinants can be used to describe the solutions of a linear system of equations, written in matrix form as $${\displaystyle Ax=b}$$. … See more Let A be a square matrix with n rows and n columns, so that it can be written as The entries See more Characterization of the determinant The determinant can be characterized by the following three key properties. To state these, it is convenient to regard an See more Historically, determinants were used long before matrices: A determinant was originally defined as a property of a system of linear equations. The determinant "determines" … See more
Determinant of a matrix wikipedia
Did you know?
WebAug 8, 2024 · Multiply this by -34 (the determinant of the 2x2) to get 1*-34 = -34. 6. Determine the sign of your answer. Next, you'll multiply your …
WebDeterminante. Der Titel dieses Artikels ist mehrdeutig. Weitere Bedeutungen sind unter Determinante (Begriffsklärung) aufgeführt. In der linearen Algebra ist die Determinante … WebJacobian matrix and determinant. In vector calculus, the Jacobian matrix ( / dʒəˈkoʊbiən /, [1] [2] [3] / dʒɪ -, jɪ -/) of a vector-valued function of several variables is the matrix of all its first-order partial derivatives. When this …
WebThe matrix determinant is a number derived from the values in array. For a three-row, three-column array, A1:C3, the determinant is defined as: MDETERM (A1:C3) equals A1* (B2*C3-B3*C2) + A2* (B3*C1-B1*C3) + A3* (B1*C2-B2*C1) Matrix determinants are generally used for solving systems of mathematical equations that involve several variables. WebAug 31, 2024 · The determinant of a triangular matrix is easy to find - it is simply the product of the diagonal elements. The eigenvalues are immediately found, and finding eigenvectors for these matrices then becomes much easier. Beware, however, that row-reducing to row-echelon form and obtaining a triangular matrix does not give you the …
WebOct 21, 2016 · 17. The determinant was originally `discovered' by Cramer when solving systems of linear equations necessary to determine the coefficients of a polynomial …
WebSep 5, 2014 · I will assume is you can reduce a matrix to row echelon form to get the aforementioned mould. This your also known as an upper triangular matrix. Calculating … cycloshow angersWebMar 29, 2024 · The trace of a square matrix is the sum of the elements on the main diagonal. Associated with each square matrix A is a number that is known as the determinant of A, denoted det A. For example, for the 2 … cycloshow atelierWebThe determinant of a matrix is a number that is specially defined only for square matrices. Determinants are mathematical objects that are very useful in the analysis and solution … cycloset copay cardWebMar 11, 2010 · The simplest way (and not a bad way, really) to find the determinant of an nxn matrix is by row reduction. By keeping in mind a few simple rules about … cycloshow fribourgIn linear algebra, a minor of a matrix A is the determinant of some smaller square matrix, cut down from A by removing one or more of its rows and columns. Minors obtained by removing just one row and one column from square matrices (first minors) are required for calculating matrix cofactors, which in turn are useful for computing both the determinant and inverse of square matrices. The requirement that the square matrix be smaller than the original matrix is often omi… cycloset manufacturerWebThe determinant is a special number that can be calculated from a matrix. The matrix has to be square (same number of rows and columns) like this one: 3 8 4 6. A Matrix. (This one has 2 Rows and 2 Columns) Let us … cycloset good rxWebApr 10, 2024 · 4/10/23, 12:50 AM Square matrix - Wikipedia 4/5 A linear transformation on given by the indicated matrix. The determinant of this matrix is −1, as the area of the … cycloset generic name